Home

More Info

  Valparaiso University, Valparaiso, IN (K-9)

  Rutgers University, New Brunswick, NJ (HS)

About Discrete Math

Prof. Dev. Home

CMSCE Home


Rutgers Home

K-8 Leadership Program

Exploring Discrete Mathematics in the Classroom

What Is Discrete Mathematics?

Discrete mathematics is a rapidly growing and increasingly used area of mathematics with many practical and relevant applications.

  • Because it is grounded in real-world problems, discrete mathematics lends itself easily to implementing the recommendations of the National Council of Teachers of Mathematics (NCTM) Standards. (The recently published "Standards and Principles for School Mathematics" notes that "As an active branch of contemporary mathematics that is widely used in business and industry, discrete mathematics should be an integral part of the school mathematics curriculum.")

  • Because many discrete math problems are simply stated and have few mathematical prerequisites, they can be introduced at all grade levels, even with children who are not yet fluent readers.

Discrete mathematics will make math concepts come alive for your students. It's an excellent tool for improving reasoning and problem-solving skills, and is appropriate for students at all levels and of all abilities. Teachers have found that discrete mathematics offers a way of motivating unmotivated students while challenging talented students at the same time.

EXAMPLE: How Many Colors?

At early elementary levels, children can color the regions on the first diagram below using different colors for adjacent regions. Eventually they can determine the minimum number of colors needed to do this and explain why this number is the minimum.

By grade 8, they can apply such coloring techniques to solve problems which involve avoiding conflicts, such as scheduling committees or final exams, devising zoo habitats, and assigning channels to radio stations.

EXAMPLE: What's The Shortest Route?

At early elementary levels, children can explore diagrams laid out on the classroom floor using masking tape (see example below). Have the children place a number of buttons on each segment to represent its length, and ask them to look for the shortest route from SCHOOL to HOME by counting the buttons along various routes.

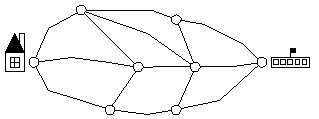
Problems involving "What's the shortest route?" can be incorporated throughout the curriculum: children can work with diagrams like this on paper (to reinforce arithmetic skills); can create their own diagrams (to reinforce measuring skills); and can solve problems based on road maps (to reinforce map-reading skills).

By grade 8, they can explore and solve more complex problems, like determining the shortest route for completing a set of errands, and can justify their solutions. For example, using the distances assigned to the lines on the diagram below, find the shortest route starting and ending at Point A, making stops at points B, C, D, and E.
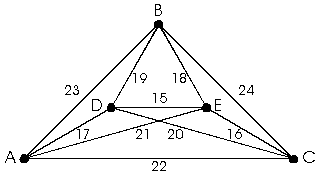
Home Page